Coaches Learn to Use Video Analysis
	Todd Allinger, Sport Science Medicine and Education Resource Center, The Orthopedic Specialty Hospital; Salt Lake City, Utah, USA

Coaches can become more skilled in the use of sport science through coaching certification programs. I have been involved in a program run by the National Coaching Institute in Canada, in which coaches learn practical skills of video analysis. In this article I'll first describe the education program. I'll then explain how we used video analysis to enhance performance in acro skiing and in synchronized swimming. Finally, I'll give practical advice for doing your own video analysis in any sport.

Coach Education
 
Canada has a strong National Coaching Certification Program that began in 1974. Over 600,000 coaches are now certified in 60 sports from Levels 1 to 5. Even at the community level, coaches are required to complete at least Level 1 before they are allowed to lead a team of kids. Certification requires a combination of classes in coaching theory (applicable to all sports), technical theory (sport specific), and practical experience (coaching).

The National Coaching Institutes (NCI) in Canada provide an environment where coaches can obtain Level 4 certification by completing 12 tasks and mentoring with a master coach in a two-year program. As an instructor for the biomechanics task at the NCI in Calgary, Alberta, I worked with some effective coaches who used their biomechanics projects to evaluate if one technique or equipment in their sport is better than another. I'll describe two studies that should stimulate other coaches to use these simple techniques.

Better Pole Length for Acro Ski Jumps
 
This study was performed by Todd Allison. Todd is Acro coach for the Canadian Freestyle Ski Association in Calgary.

Inside jump showing the approach (top), right ski edge set and pole plant (middle), and flight after 360 degrees of counter clockwise rotation (bottom). Skier is using the short pole grip (left hand). Markers at the lower corners in pictures identify the fall line (down hill to the left).

Acro skiing, formerly known as ballet skiing, incorporates jumps that make up a large portion of the score (25 - 50%). These jumps are executed on smooth terrain by planting the ski poles, thrusting upwards with legs and arms, rotating about the long axis of the body (twisting), and landing on the skis. An athlete is scored on both the cleanliness of their jump as well as the amount of rotation (related to jump height) that is achieved.

Ski poles are used to vault the athlete during a jump, so Todd set out to determine how the height of the pole grip above the snow affected performance. The rule of thumb was that the ski pole should be long enough so that when it is planted in the snow and the grip is held normally, the extended arm is parallel to the ground.

Ten national team skiers (from four countries) performed 10 jumps: 5 with their traditional length poles and 5 with a lower pole grip (short), which allowed for a 90 degrees bend of the elbow at take-off. An 8-mm video camera recorded the jumps at a right angle to the take-off location, approximately 18 meters away. Two markers placed on the fall line indicated the take-off area. Frames of video were selected that represented the take-off, the peak height of the jump, and the landing. The take-off boot was digitized manually for the three selected frames using a personal computer with a frame grabber. The number of pixels recorded from digitization were converted to distances in meters after digitizing a video frame that had a meter stick that was held in the take-off area.

Most skiers thought they could jump higher if the pole was gripped higher, similar to pole vaulting. However, the skiers jumped 5.5 cm (11 %) higher on average with the shorter pole grip than with the traditional length pole. Apparently, a lower grip allowed the shoulder and elbow joints to provide more downward impulse than when the pole was gripped higher. An increase in jump height of this order should improve the judged score.

Better Technique for Continuous Spin Scull in Synchro Swimming
 
This project was carried out by Erin Gillings of the Aquabelles Synchronized Swimming Club, Calgary. She addressed the question of which technique is better for the continuous spin scull in synchronized swimming: split scull or double-overhead scull.

Side view (A) and bottom view (B) of the split scull (left) and double-overhead scull (right) techniques.

The split scull is the most popular method used in the sport, but the athletes often have a tilted body position while spinning. The double-overhead scull may alleviate the tilt in the body, but may reduce the height that the legs project above the water.

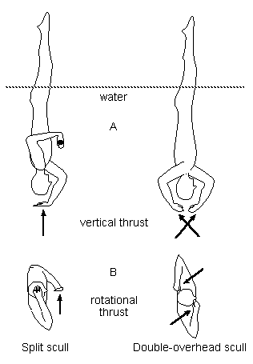In the continuous spin scull, the athlete projects the legs vertically above the water and spins while the body is slowly lowered into the water. From the judges' perspective, the key elements are the initial height of the legs above the water, the vertical alignment, the number of spins before the feet are submerged, and a constant revolution speed. In the split scull, one hand sculls above the head to provide vertical thrust and the other hand sculls in front of the chest to rotate the body. In the double-overhead scull both hands are above the swimmer's head and provide vertical thrust and rotation, depending on the angulation of the hands while sculling.

Three Olympic silver medalists (1996, Atlanta) from the Canadian synchronized swimming team performed four continuous spins: twice with the split scull and twice with the double-overhead scull techniques. The athletes were video taped (30 frames/second) from an underwater viewing window. The coordinates of the pubic bone and the surface of the water between the legs were digitized for every 180 degrees of body rotation. From the digitized points the height of the toes above the water, leg tilt angle, spin rate, and drop space were calculated.

Results from this video analysis give strong evidence that the double-overhead technique is superior to the split scull technique for the continuous spin scull. The double-overhead scull produces greater height (5.1 cm and 5.0 cm higher for two of the three athletes), more rotations (6.5 vs 4.6 revolutions), and a smoother descent into the water than the split scull.

Biomechanically, the double-overhead scull should produce a better vertical alignment and less motion about the axis of the spin than the split scull. The double-overhead technique creates a pure moment around the axis of rotation. With the split scull technique, the hand in front of chest produces the spin but is not counter balanced by another force on the other side of the body. As a result, upper body contortions below the water are required to keep the legs vertical. The result is less leg height and fewer revolutions.

How to do Video Analysis
 
Both of these studies answered an important performance question through measurements taken from a video. If coaches simply view tapes--so-called qualitative analysis--they might miss changes in performance. The same video tapes used for qualitative analysis can be used for quantitative evaluations if a few simple steps are followed.

First, the procedure for collecting video data: 

1. Mount the camera in a fixed position on a rigid tripod.
2. Place the axis of the camera perpendicular to the plane of motion, as shown in the figure below.
3. Place the camera as far away from the motion as is practical to avoid parallax errors.
4. Zoom the lens so the action fills the field of view as much as possible without cutting out critical body parts.
5. Level the camera using a bubble level on the tripod or camera.
6. The center of the field of view (height and width) should be at the center of the desired motion.
7. Focus the lens.
8. If the camera has an adjustable shutter, reduce the exposure time to as short of a period as possible without compromising the picture brightness. Extra lighting may be required. A short exposure time reduces the blurring of a fast motion when a video frame is paused.
9. Display the date and time on the camera, if possible. This info helps to reduce digitizing time and helps keep track of data.
10. Record a few frames of a meter stick held in the plane of motion. Use these frames to calibrate the data after digitization.

Top view of camera setup.

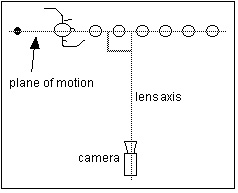Frame showing meter stick to be digitized for calibration.

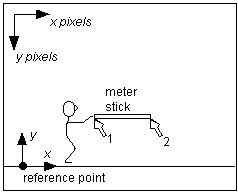You get data for the positions of objects by digitizing the video. An example of a shotput flying through the air is shown.

Series of 7 frames of the flight of a shot put. Frame 0 is just after release from the hand. The position of the shot in every 10th frame is shown.

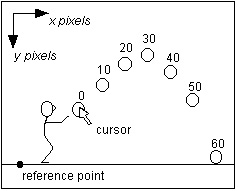Follow these steps for digitizing with a computer :  

1. Connect the VCR or video camera to a frame capture card in the computer.
2. Pause the video tape on the frame to be digitized.
3. Capture the video frame. The picture is now shown on the computer screen.
4. Digitize the points of interest by placing the cursor over the center of mass of the object and pressing the mouse button. Also, make sure to digitize a stationary reference point. This may be done in any program that shows the location of your cursor on the screen in pixels (e.g., a drawing, painting, or imaging program).
5. Advance the video one or more frames and repeat the digitization of the points (step 4).
6. Digitize a meter stick that was held in the plane of motion. This calibration frame is used to determine the number of pixels in a meter.
7. Save the data in an ASCII file. The x, y coordinates (pixels) of each point are now stored for each frame. When the data are opened or copied into a spread sheet it will resemble the table shown below. Every 10th frame was digitized:

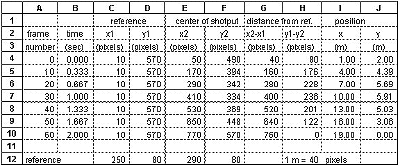
If no computer is available, the video can be digitized directly from the television screen as follows:

1. Connect the VCR or video camera to a television monitor.
2. Pause the video tape on the frame to be digitized.
3. Place a clear plastic sheet over the television monitor. Make sure the sheet does not slide on the screen.
4. Use a felt pen to mark the points of interest. Make sure to digitize a stationary reference point.
5. Advance the video one frame and repeat the digitization of the points (step 4).
6. Digitize a meter stick that was held in the plane of motion for calibration. See the figure below.
7. Using a ruler, measure the x and y distance (mm) from the reference point to the point of interest. Write these down or store them in a spread sheet on the computer for each video frame.

Now you can convert the digitized points (pixels) or the distances measured off the screen (mm) into true distances:

1. Subtract the digitized points from the reference point to make it the new origin (see figure above and table below). Point 1 is subtracted from point 2 in the x-direction. Point 2 is subtracted from point one in the y-direction to reverse the sign of y from the screen (origin in upper left corner) to the ground based coordinate system (origin at reference point).
2. Determine the number of digitized pixels there are in 1 m (x2 - x1 = 290 - 250 = 40 pixels = 1 m).
3. Divide each data point by the number of pixels in 1 m. Now the digitized points are calibrated to distance of the original action.

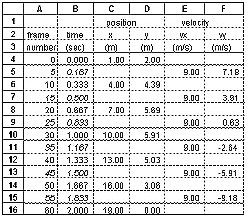
The velocity of a point can be calculated from the position data, because the average velocity between two points equals the change in distance divided by the change in time:
     v = (p2 - p1) / (t2 - t1). Here's how to do it:

1. Subtract the second data point (p2) from the first (p1).
2. Divide this value by the change in time (t2 - t1). A typical 60 Hz video camera records 30 frames / second. If your VCR can display fields, you will have 60 fields / second (two fields combine to make one frame).

Velocity is calculated here as an average from one digitized frame to the next. Therefore, the time related to this velocity is midway between the two digitized frames. For example, the velocity of the shotput in the x direction at time = 0.167 s is:
     vx5 = (px10 - px0) / (t10 - t0) = (4.0 - 1.0) / (0.333 - 0.0) = 9.00 m/s
Here are the calculations of velocities in the x and y directions for the data in the above table:

For more information on calculating angles and accelerations consult a source such as Hay (1985).

Reference

Hay, J.G. (1985).The biomechanics of sports techniques. Prentice-Hall: Englewood Cliffs, NJ.